Formal Gear Strength Analysis

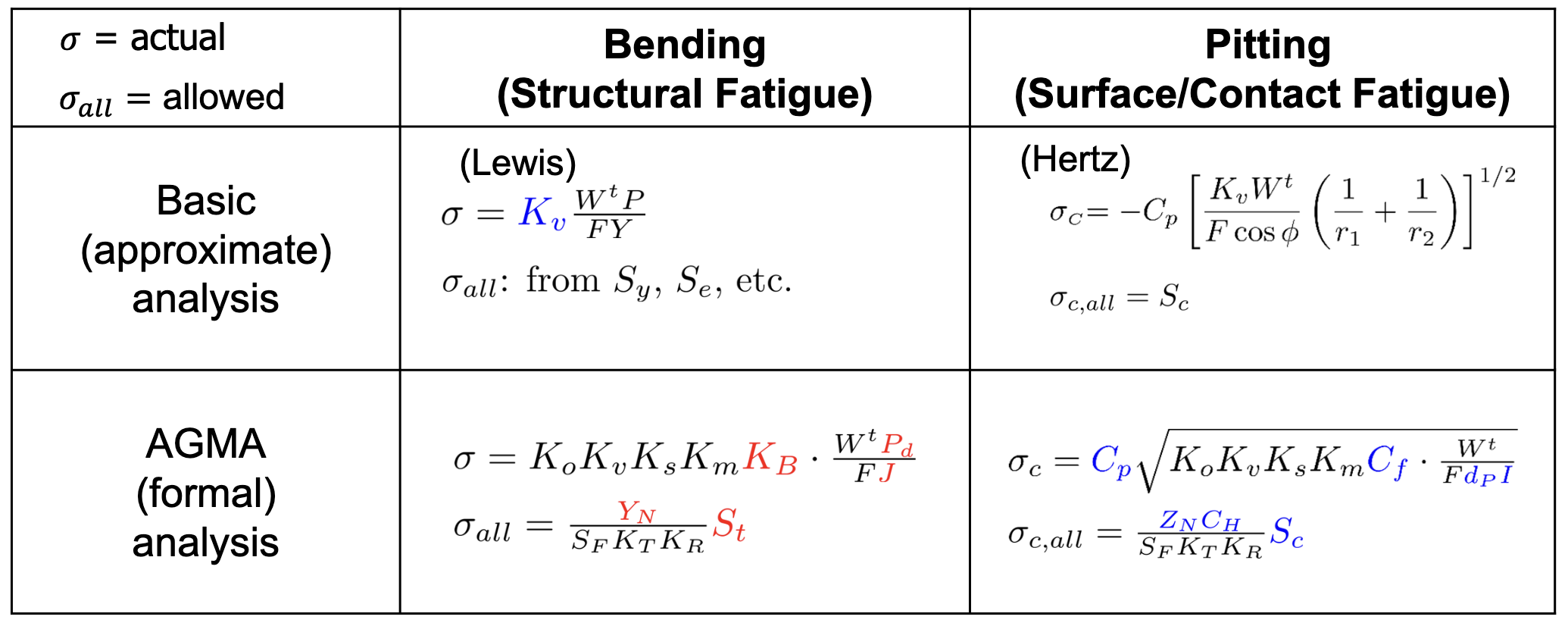
AGMA Equations

The Lewis and Hertz equations for Selecting Gears are basic techniques which are applicable to spur and helical gears only. These are rudimentary and preliminary estimates.

The AGMA equations are the real deal…
The fundamental equation for actual bending (fatigue)

• ${\sigma }_G=K_o{K}_v{K}_s{K}_m{K}_B\frac{w_{tg}{P}_d}{F{J}_G}$ The fundamental equation for actual pitting (contact)
• ${\sigma }_c=C_p\sqrt{K_o{K}_v{K}_s{K}_m{C}_f\frac{w^t}{F{d}_{p}I}}$ Where these coefficients are…
• $K_o$: overload factor
• $K_v$: dynamic factor
• $K_s$: size factor
• $K_m$: load distribution factor
• $K_B$: rim thickness factor
• $d_P$: pitch diameter of pinion
• $C_P$: elastic coefficient which comes from here
• $C_f$: surface condition factor
• $J,I:$ geometric factors
  • You can find J from charts, by using the number of gear teeth of the x-axis (pinion axis), and the curves (gear-curves), find the intersection and then J is on the y-axis. These factors would come from tables…

Allowable Bending Strength

${\sigma }_{all}=\frac{Y_N}{S_F{K}_T{K}_R}{S}_t$

Allowable Contact Strength

${\sigma }_{c,all}=\frac{Z_N{C}_H}{S_F{K}_T{K}_R}{S}_c$

Where the coefficients here are…

• $S_F$: safety factor
• $K_T$: temperature factor
• $K_R$: reliability factor
• $Y_N$: stress-cycle factor (for bending)
• $Z_N$: stress-cycle factor (for pitting)
• $C_H$: hardness ratio factor

AGMA Strength Equations

Equation for allowable bending strength
${\sigma }_{all}=\frac{Y_N}{S_F{K}_T{K}_R}{S}_t$
Equation for allowable contact strength
${\sigma }_{c,all}=\frac{Z_N{C}_H}{S_F{K}_T{K}_R}{S}_C$

• This is just adding familiar correction factors

Digging into coefficients

Elastic Coefficient

• $C_p={\left[\frac{1}{\pi \left(\frac{1-v_p^2}{E_p}+\frac{1-v_g^2}{E_G}\right)}\right]}^{\frac{1}{2}}$

Geometric Factors (J and I for bending and surfaces)

• You get these factors from graphs, given a pressure angle and using the number of gear teeth of the x-axis (pinion axis), and the curves (gear-curves), find the intersection and then J is on the y-axis.
• For helical gears, you then multiply J by an F-factor based on the amount of mating teeth which comes from another chart

Dynamic Factors

$$\{\begin{array}{llc}{\left(\frac{A+\sqrt{V}}{A}\right)}^B,& V\text{ in ft/min}& A=50+56(1-B)\\ {\left(\frac{A+\sqrt{200V}}{A}\right)}^B,& V\text{ in m/s}& B=0.25(12-Q_v{)}^{2/3}\end{array}$$

Size Factor

• The size factor, $K_s$ is typically 1
• Sometimes $K_s=1.192{\left(\frac{F\sqrt{Y}}{P}\right)}^{0.0535}$

Rim Thickness Factor
$K_B=\{\begin{array}{ll}1.6\ln \left(\frac{2.242}{m_B}\right),& m_B<1.2\\ 1,& m_B\ge 1.2\end{array}$
Where $m_B$ is the backup ratio here…

Reliability Factor $K_R$

• This tells us what % of gears should survive N cycles…

$K_R=\{\begin{array}{ll}0.658-0.0759\ln (1-R),& 0.5<R<0.99\text{Medium reliability}\\ 0.5-0.109\ln (1-R),& 0.99\le R\le 0.9999\text{Ultra-high reliability}\end{array}$
Temperature Factor $K_T$

• Will always be 1 in MTE 322 - Electromechanical Machine Design but there are equations for higher temperatures

Other Factors

• $C_H$, $Y_N$, $Z_N$ can be obtained from the Shigley tables…

Strength Analysis Overview